The Mechanics Of A Breaking Pitch

The Physics Of A Curveball
By Professor Peter J. Brancazio,
Department of Physics,
Brooklyn College,CCNY
NYC, NY

For years, many scientists believed that the curveball was an optical illusion. As we shall see, this is not true. In fact, physicists have long been aware of the fact that a spinning ball curves in flight, going back to Isaac Newton, who wrote a paper on the subject in 1671. In 1852, the German physicist Gustav Magnus revived the topic when he demonstrated in an experiment that when a spinning object moves through a fluid it experiences a sideways force. This phenomenon, now known as the Magnus Effect, is the fundamental principle behind the curved flight of any spinning ball.

The theory of the Magnus Effect is a relatively simple exercise in aerodynamics. When any object is moving through the air, its surface interacts with a thin layer of air known as the boundary layer. In the case of a sphere, which has a very poor aerodynamic shape, the air in the boundary layer peels away from the surface, creating a "wake" or low-pressure region behind the ball. The front-to-back pressure difference creates a backward force on the ball, which slows its forward motion. This is the normal air resistance, or aerodynamic drag, that acts on any object moving through the air. However, if the sphere is spinning as it moves, the boundary layer separates at different points on opposite sides of the ball–further upstream on the side of the ball that is turning into the airflow, and further downstream on the side of the ball turning backward. As a consequence, the air flowing around the ball is deflected slightly sideways, resulting in an asymmetrical wake behind the ball. The effect is to generate a pressure difference across the ball, creating a lateral force component that pushes the ball sideways. This lateral force, at right angles to the forward motion of the ball, is known as the Magnus force.

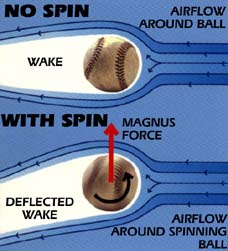
The strength of the Magnus force is in direct proportion to the rate of spin as well as the forward speed of the ball–the greater the forward speed, the greater the force. It will also be proportional to the air density, which means that a ball will tend to curve less at higher altitudes where the air is thinner–a boon to hitters in a high-altitude city like Denver. The stitches on the baseball also help to increase the Magnus force–not only by increasing the thickness of the boundary layer, but also by providing a place for the pitcher to put his fingers so that he can put more spin on the ball. It should be noted, however, that stitches are not required to make a ball curve. Even a smooth-surfaced table tennis ball will curve if it is given enough spin.

On the other hand, the direction of the Magnus force depends only on the direction of spin. As shown in the diagram, the force is always directed toward the side of the ball that is turning backward. In other words, the Magnus force always points in the same direction that the front of the ball is turning toward.

By properly orienting the spin direction, a pitcher can make the Magnus force point in any direction–left, right, up, down and so on. For example, the natural clockwise rotation of a righthander's wrist creates a leftward force (from the pitcher's perspective), which causes the ball to curve away from a righthand batter. When thrown with a three-quarters overhand motion, the same pitch will curve down and away from the batter. Conversely, a lefthand pitcher's natural wrist rotation (which is counterclockwise) causes the ball to curve left to right–that is, into a righthand batter and away from a lefthander. In order for a righthand pitcher to imitate this motion of throwing the pitch known as a screwball, he must turn his wrist counterclockwise as he releases the ball–an unnatural, uncomfortable motion that frequently leads to elbow trouble. Much of the strategy of baseball is a direct consequence of the fact that righthanders and lefthanders throw different pitches, simply because of the quirk of human physiology that our hands rotate more easily in one direction than the other.

A ball can be made to curve in a vertical plane as well. In fact, the trajectory of any thrown pitch has a natural downward curvature due to the force of gravity. However, by changing the spin direction, a pitcher can increase or decrease the curvature. For example, when a ball is thrown with a topspin, the Magnus force will act toward the ground, causing it to curve more sharply. If the ball is thrown with a backspin, the Magnus force will point away from the ground, causing the ball to curve less. The latter pitch produces what is often called a rising fastball. However, the laws of aerodynamics tell us that for a baseball to physically rise (that is, curve upward) as it approaches the batter, the Magnus force would have to be greater than the weight of the ball, and the rate of spin required to generate this much force is far beyond the ability of any pitcher. Thus, the rising fastball is an optical illusion. The baseball simply falls less than the batter expects it to.

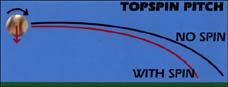
In these two illustrations, the Magnus Force is indicated by the red arrows.

It seems pretty clear that no right-minded physicist would ever argue that a curveball is an illusion. However, as with the case of the rising fastball, we will argue that the sharp break of a curveball is illusory. While many hitters often report that a good overhand curveball breaks so sharply that it looks like it is falling off a table, the laws of aerodynamics clearly show that the Magnus force cannot suddenly increase in flight–as would be required for a sudden change in curvature–but can only get smaller as the spin and speed of the ball slow down. The explanation for this illusion has to do with how the batter perceives the flight of the ball. The angular motion of the ball–that is, its apparent motion across the batter's field of vision–seems relatively slow at first, but then increases rapidly as the ball approaches. In fact, it has been demonstrated that the angular motion becomes so rapid that no batter could possibly move his head fast enough to keep his eye on the ball all the way. When a good curveball is thrown, the change in its angular motion becomes even more pronounced as it nears the batter, greatly enhancing the appearance of its natural curvature and giving the illusion of a sharp bend.

The Mechanics Of A Breaking Pitch USAToday.Com (1993). Retrieved December 4, 2005, from USA Today: http://www.usatoday.com/weather/wdensity.htm